Stigmergy versus behavioral flexibility and planning in honeybee comb construction Stigmergy versus behavioral flexibility and planning in honeybee comb construction

Perhaps the most magnificent animal building structure is the honeybee wax comb—a double-sided sheet of tessellated, near-horizontal hexagonal cells. The cells are built either side of a common backplane that forms a base for those to either side. This typically highly regular structure has been shown to be mathematically optimal to maximize storage space and stability while minimizing building material (1). However, Smith et al. (2) show that bees also build various types of irregularly shaped and sized cells, for example when merging separate comb constructions. This raises the question of whether the bees’ innate behavioral repertoire contains multiple different routines for each shape, whether bees plan ahead to insert optimal shapes, or whether such diversity of structures could be explained by simple rules.

The hexagonal grid structure of honeycomb, constructed by a leaderless collective of hundreds of bees, lends itself to speculation that a robotic, repetitive innate behavior routine must be at work. An analogy is the construction of a brick wall, where each new layer is built by adding new bricks in a staggered, one-over-two pattern. This can be efficiently achieved by a robot without the architect’s supervision (3). This concept—where the features of an existing structure are used to add the next element of the structure by a simple rule—is called stigmergy (4, 5). The perceived analogy between insect cells and bricks has led some social insect researchers to model comb construction as the simple process of fitting new, complete comb cells onto the existing structure (5).

However, a hexagonal cell is not an externally supplied prefabricated unit. Rather, the bee builds a cell using small specks of wax which are chewed, deposited, and sculpted into the walls (6) to form a hexagon of equal-length sides with internal angles of 120°. And this is not the only challenge where the analogy with the brick-laying robot fails.

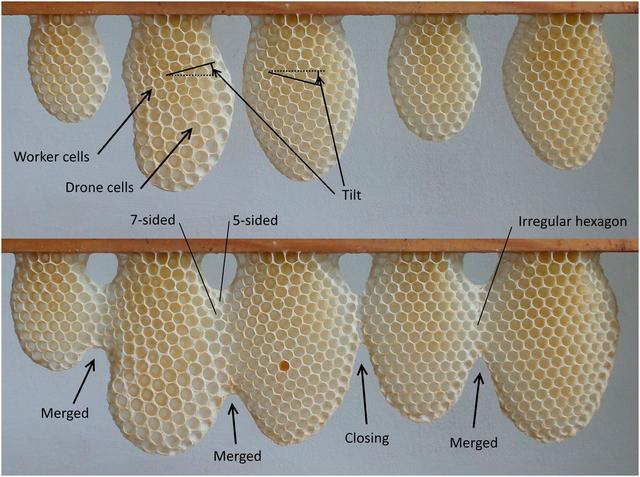
The comb of honeybees is usually built downward, attached to the underside of a supporting structure such as a tree branch, a rocky outcrop (in open-nesting honeybee species), or the upper surface of a cavity such as a hollow tree (in cavity-nesting species such as the familiar western honeybee, Apis mellifera, the species under study here), and none of these natural surfaces are flat and smooth (7). The behavior must therefore be sufficiently flexible to cope with additional complexities such as slopes, protrusions, and fissures. One might assume that once the foundation is laid, bees could then switch to simple rules, building repetitive hexagons whose size is aligned with the worker’s body dimensions—but they must also build hexagonal drone cells that are 1.2 times larger than worker cells. A transition (the change from one size to the other) necessitates yet further complexity of the hypothetical building rules. Finally, a blade of honeycomb will typically begin as several individual teardrop-shaped tongues (8) (Fig. 1). As they are extended, they come into contact so the bees must adapt the cell structures to merge those of differing size, orientation, and offset. Smith et al. (2) explore how bees cope with these many challenges and ask whether comb-constructing bees can be regarded as automatons or architects.

The authors obtained measurements of both regular and irregular cells. This was achieved using a combination of image-processing methods for edge detection, allowing their software to locate cell walls. Further software then performed the geometric calculations to locate cell centers and vertices and to extract cell metrics such as area and wall lengths. The advantages of both human and automated processing were combined so inaccuracies that are typical of automated image processing were subsequently removed by human editing. Automation has allowed the authors to measure more than 12,000 cells and to investigate the adaptations and compromises made by bees when confronted by the need to change or to unite discrete sections of regular honeycomb. Areas of interest that were studied included the transitions from worker to drone cells and the merging of sections that involved different cell alignment, tilt (orientation of the hexagon’s vertices), and size (worker and drone). These manually defined zones were arranged to span 30 mm (five or six cells) either side of an interface (transition or merger), thus sampling both regular and irregular cell formation. An enormous diversity of cell shapes was found: Pentagons and heptagons were the most commonly observed nonhexagonal shapes, but there were also four-, eight-, and nine-sided cells.

Smith et al. (2) show that adaptations between areas of cells of differing tilt requires smaller adjustments where the cells are more closely aligned, hence a progressive change of tilt to match one with another should result in reduced irregularity and fewer nonhexagonal cells. While there is a greater variation of both wall length and the number of walls per cell within an interface zone, the distribution remains bimodal, aligning well with the values typical of either drone or worker cells (2). The most common nonhexagonal cells (pentagons and heptagons) were often found in pairs or triplets, where a cell with a surplus face was found adjacent to with another with fewer. It appears that bees, even when prevented from building a perfect specimen, will deviate from that ideal by as little as possible.

An interface between worker and drone cells can result from a transition or a merger of two construction sites, both of which introduce irregular cells with variations in both area and wall length. The analysis (2) shows a distinction between the controlled transition between worker and drone combs, compared with the less-coordinated merger between two tongues of downward-growing comb. In the former case, the cells within the interface were found to change progressively from one size to the other and resulted in few that were too small, while merge zones contained a wider spread of size with many less usable, smaller cells. This suggests that bees were more successful in adjusting the cell size when matters were under their control than when presented with two disassociated combs.

For each class of merger (changes of size, displacement, and rotational misalignment), the authors show the degree of irregularity at locations across the junction. Most of the irregular cells are concentrated within a distance of 15 to 20 mm, or two or three cells. The authors note that this range, the distance over which the adaptation takes place, is less than the foot-to-foot span of a honeybee. Therefore, a comb builder would be able to sense the walls and corners of cells on both sides of an adaptive zone and so she could detect both the arrangement of the comb onto which she will build and that of the required outcome. With input from both arrangements balancing any deviations from the ideal length, angle to others, and distance from an opposing cell wall, she can find a cell vertex on each side of the gap to be connected by a suitable wall.

It would appear, therefore, that bees have a repertoire of techniques which by default will build regular cells of a set size yet can also build regular cells of a different size, graduate from one size to the other, merge mismatched cells, cope with irregular foundation, and generate curved architecture through lateral asymmetry (9, 10). Furthermore, it appears that the technique chosen for a particular adaptation is well-suited to the task as few cells are produced that are distorted to the point of being unusable (2). Cells that are of an incorrect size or not hexagonal will seldom be used to raise brood but will be utilized for food storage and so are not wasted.

What might explain the diversity of seemingly intelligent solutions to such varied geometrical building challenges? One possibility is a combination of stigmergy combined with a highly rich behavioral repertoire—with one preprogrammed rule for each cell type (hexagonal cells for workers and drones, heptagons, pentagons, etc.). One might then postulate that each routine is triggered by one geometric constellation of existing wax structures. However, a suitably complete set of instructions becomes somewhat burdensome, as expressed by Donald Griffin (11): “Environmental conditions vary so much that for an animal’s brain to have programmed specifications for optimal behavior in all situations would require an impossibly lengthy instruction book.”

A cognitive explanation for the observed diversity of solutions is that bees might have a form of mental template of the desired outcome of the construction (9, 10), assess the existing geometry, and then decide whether building an irregularly shaped hexagon, or perhaps a pentagon or heptagon as a compromise, is the optimal solution. Such forward planning is implied by Smith et al. (2) in the transition from standard worker to larger drone cells: The authors observe that workers, in preparation for the construction of fully sized drone cells, will build intermediate-sized cells—as if they were aware of the next phase of their construction and taking preemptive steps to facilitate the transition. However, it appears equally possible that the intermediate-sized cells arise from simple rules—if three sides of the construction are formed by worker comb cells, then adding three more sides of typical drone cell dimensions will generate an intermediate-sized cell.

So, perhaps we ought not to dismiss the notion of stigmergy just yet—we simply need to examine it at a finer-grained level than the addition of entire comb cell shapes (the bricklayer robot analogy above). Assuming that stigmergy is the mechanism that causes social insects such as bees to work without a guiding mind but collectively toward an architectural goal, then the next step would be to characterize the repertoire of elementary tasks, i.e., building a wall in an optimal direction relative to existing cell walls, and of a required length. The structure of a cell is an emergent property of these actions, and a cell is the result of the construction of a set of walls. If the walls of an existing structure are perfectly built, then a perfect cell can easily be added to this structure. Where the requirement is more of the same, the rule could be simply to build a standard-sized wall at 120°.

If the current state is irregular, or the required outcome diverges from the current form, then the task would be slightly different, perhaps some parameterized version of a basic building of building walls at 120°. Misaligned walls from two neighboring construction sites will inevitably result in an irregular cell, no matter how the vertices of neighboring cells are connected with a new wall. Considering the construction of a wall to be an elementary task removes the need for the bees to have the specific ability to build a hexagonal cell, and one with five sides, and one with seven sides. Furthermore, knowing how to build walls of approximately the right size eliminates the need for knowledge of how to build worker-sized cells, and drone-sized ones, and those of an intermediate size.

Stigmergy has its origins in the study of social insects (4, 12), but it has more recently been embraced by roboticists and space engineers (13, 14). The latter consider that stigmergy can contribute to the design of systems for extraterrestrial construction in orbit or elsewhere. Communications delays, due to the vast distances, eliminate the possibility of direct control from the ground, and hence local decision-making is desirable. Overall reliability is enhanced by distributed processing as this reduces the potential for a single point of failure. Although there would be no overall controller, the goals could be achieved by the members of a robotic workforce operating as autonomous units, each with a repertoire of possible actions. A specific action would be chosen based on whatever needs to be done together with the current state of the construction/workpiece. Using cleverer forms of stigmergy than the simple bricklaying approach would be essential, however: A swarm of bricklaying robots released on an alien planet would fail at the hurdle of making bricks. Even if provided with these, and the basic one-over-two rule for building brick walls, they might build impressively large but not necessarily useful constructions. Studies such as Smith et al. (2) provide useful input to the question of how much complexity can be achieved by simple rules, and the extent to which these might have to be paired with some form of blueprint of the desired outcome to generate functional constructions.